Pilot-wave hydrodynamics
In 2005, Yves Couder and Emmanuel Fort discovered that droplets walking on a vibrating fluid bath exhibit features previously thought to be peculiar to the quantum realm. Since 2010, this hydrodynamic system has been the focus of my group’s research. Particular attention has been given to identifying new hydrodynamic quantum analogs in the laboratory, and to rationalizing the emergent quantum-like behaviour theoretically. We have also developed and explored theoretically a broader class of pilot-wave systems that provide a mathematical bridge between the walking-droplet system and the dynamics proposed by Louis de Broglie and others for the microscopic, quantum realm. For an overview, I suggest reading the reviews on the subject, especially the most recent one, Hydrodynamic Quantum Analogs, as appeared in 2020 in Reviews of Progress in Physics.
- Hydrodynamic quantum analogs. ROPP, 2020.

- Introduction to HQA focus issue. Chaos, 2018.

- Pilot-wave hydrodynamics. ARFM, 2015

- The new wave of pilot-wave theory. Physics Today, 2015.

Bouncing and walking droplets:
towards a hydrodynamic pilot-wave theory
Our first studies were focused on understanding the dynamics of droplets bouncing and walking on a vibrating bath. Progress was made by virtue of an integrated experimental and theoretical program. Experimental advances enabled precise drop generation, bath vibration and flow visualization. Theoretical developments have given rise to a robust stroboscopic model that provides a quantitative description of free walker dynamics. Subsequent theoretical refinements have allowed for an accurate description of more complex walker system, including multiple-walker system, and walker interactions with submerged boundaries.
- The stability of droplet pairs

- Crossing the Faraday threshold

- Surface Schlieren and walking drops
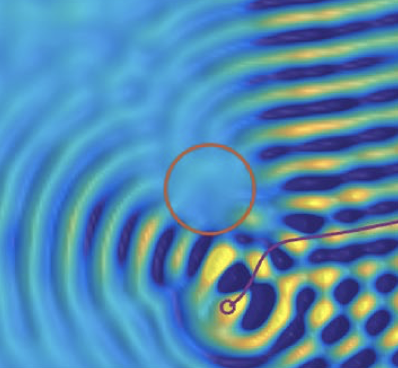
- Non-specular reflection
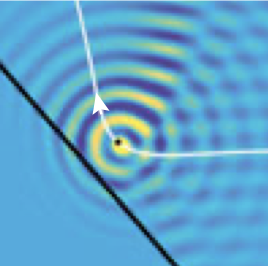
- Visualizing pilot-wave phenomena

- Building a better shaker table

- Building a better drop generator

- Faraday pilot-wave dynamics

- The stroboscopic model

- Bouncing and walking exotica
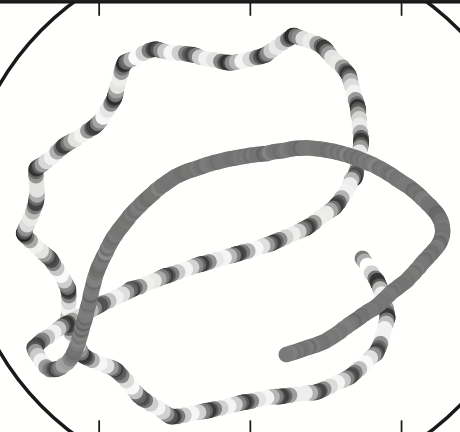
- Walking droplets: theory

- Bouncing droplets: theory
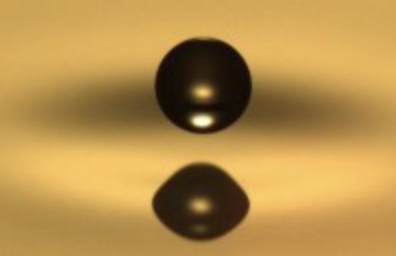
- Quasi-static drop impact
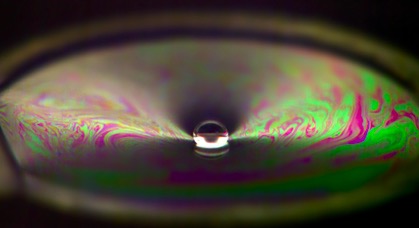
- Drops bouncing on a vibrating soap film
Hydrodynamic Quantum Analogs
The second focus of our research has involved an examination of the potential and limitations of the walking-droplet system as a quantum analog. We began by revisiting a number of the early hydrodynamic quantum analogs (HQAs) discovered by Yves Couder, Emmanuel Fort and collaborators in Paris, including walker diffraction, orbital walker dynamics, crystal vibrations and walker tunneling. We have also discovered and explored a number of new HQAs, including walkers in corrals, Friedel oscillations and spin lattices. Likewise, we have examined a number of new hydrodynamic analogs of optical systems, including analogs of the Talbot effect and optical dimers. Our work has demonstrated that the mean pilot-wave potential can play a role analogous to the non-local quantum potential in Bohmian mechanics and so makes clear how classical, local pilot-wave dynamics can give rise to apparently nonlocal behaviour.
- Hydrodynamic spin lattices
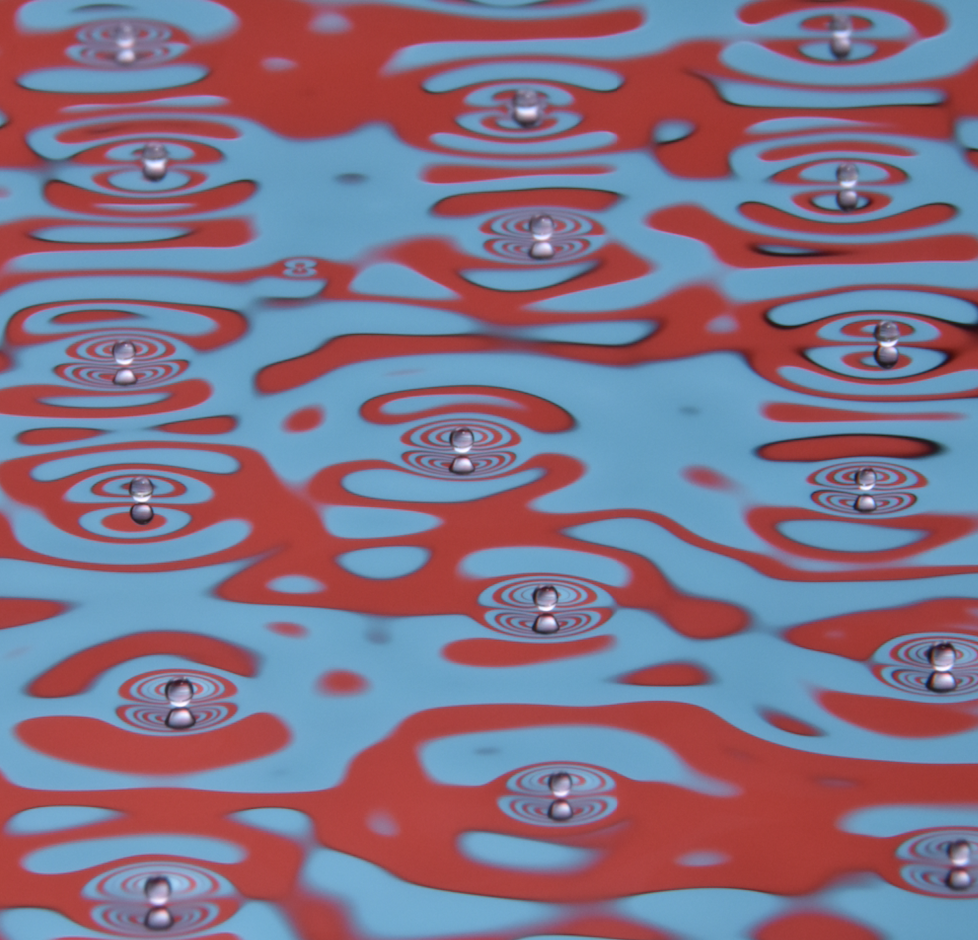
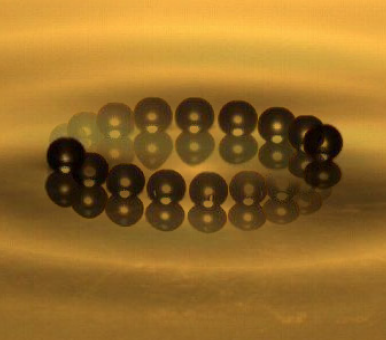
- Bouncing drops: free rings

- HQA of Friedel oscillations
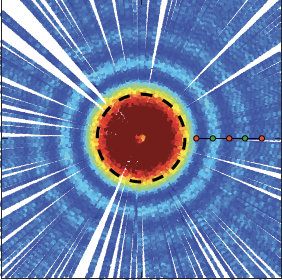
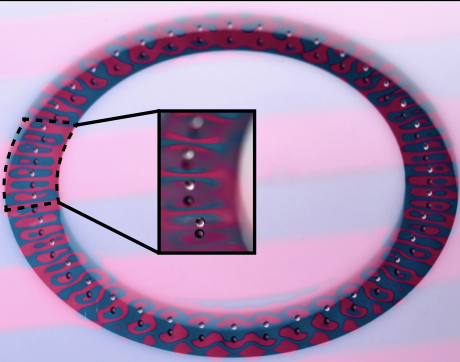
- Bouncers in a confined ring
- Walkers tunnel: experiments
- Ratcheting droplet pairs
- The mean-pilot-wave potential
- Orbits in a circular corral
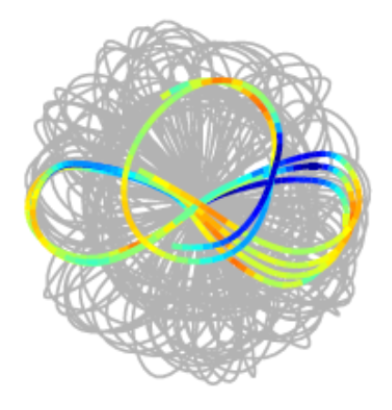
- Walker-pillar interactions
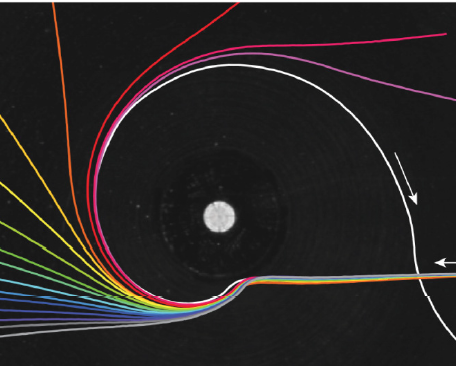
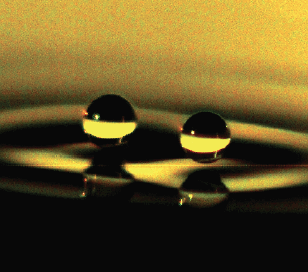
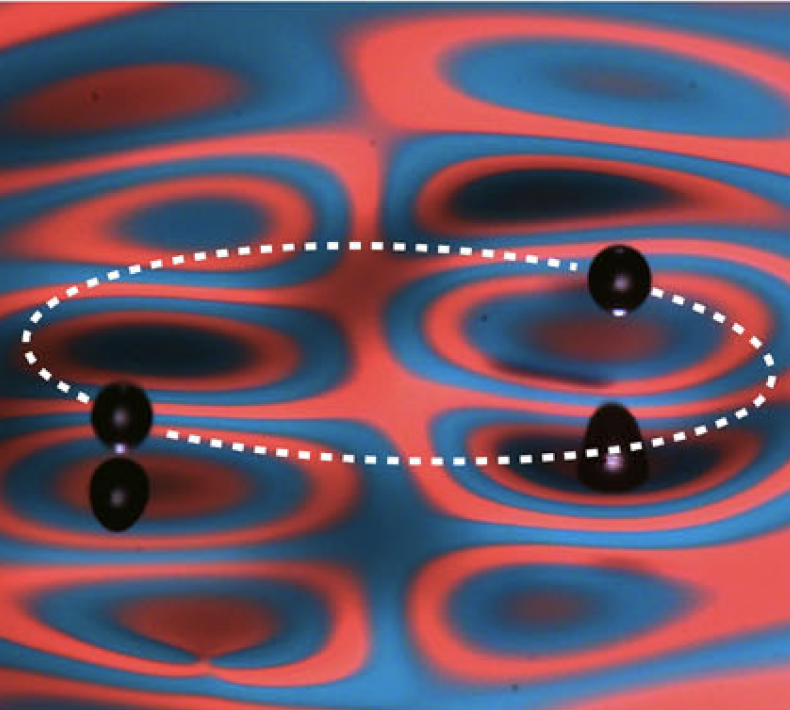
- Promenading droplet pairs

- Walker motion in a spring force

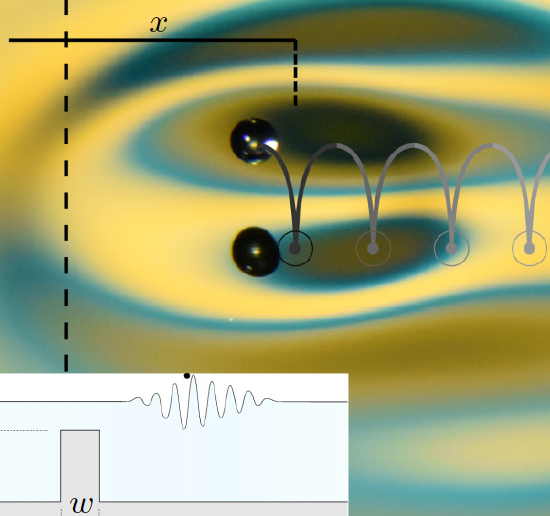
- Walkers tunnel: numerics
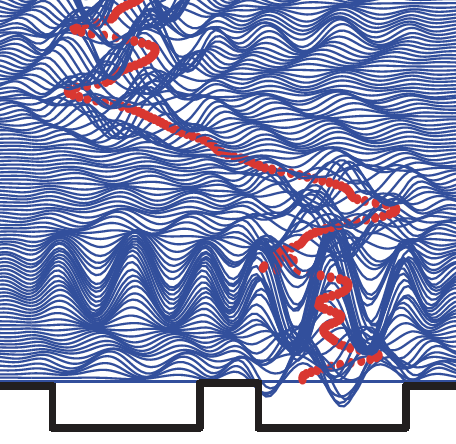
- Walker slit diffraction
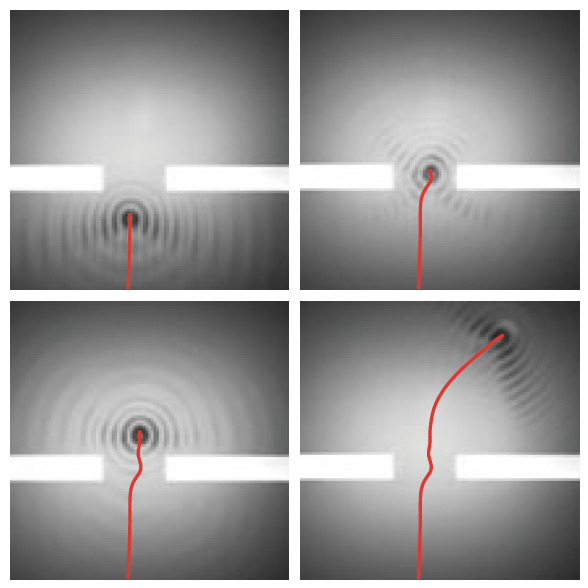
- Orbiting droplet pairs
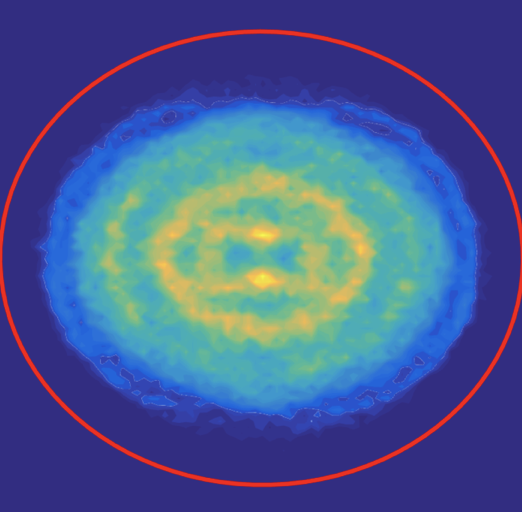
- Statistical projection effects
- Hydrodynamic Talbot effect

- Circular orbits in a central force
- Hydrodynamic boost factor

- Exotic orbits in a rotating frame
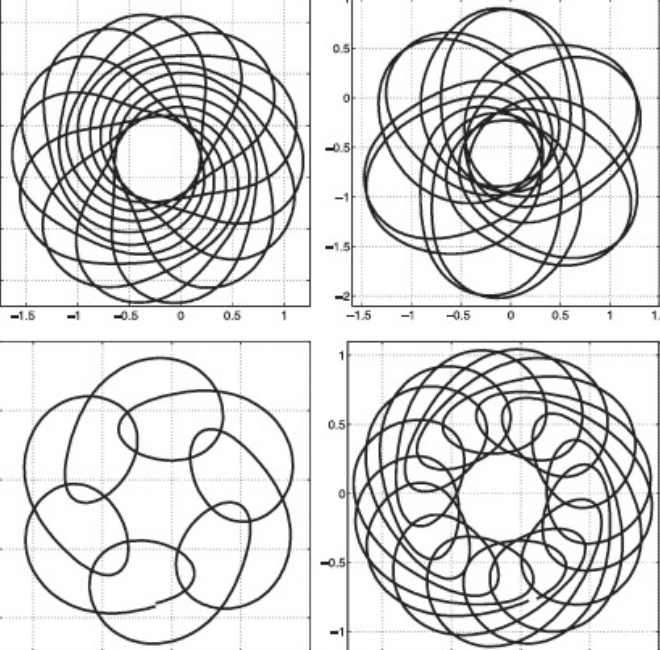
- Rotating frame: Orbital stability
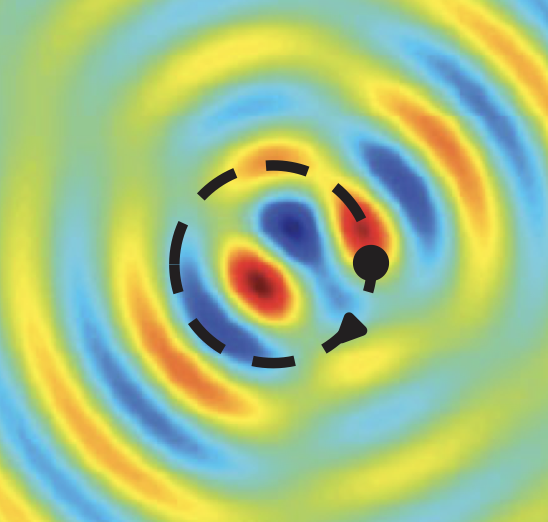
- Rotating frame: Experiments

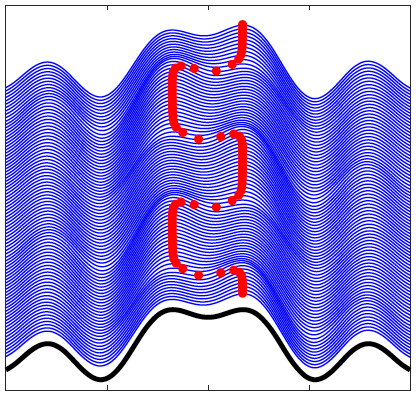
- An HQA of the quantum corral
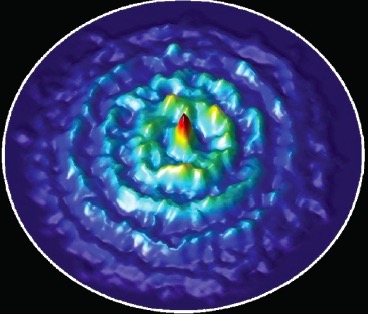
- Video: Pilot-wave hydrodynamics

- QM writ large

The Generalized Pilot-Wave Framework
The key dynamical features responsible for the quantum features of the walking-droplet system are the resonance between the particle and the wave, and the concomitant lingering quasi-monochromatic pilot-wave field. Having identified these key features, we may proceed by exploring a broader class of dynamical systems with these features. Doing so has revealed the possibility of capturing additional quantum features with classical pilot-wave systems. The generalized pilot-wave framework also provides a basis for comparing the walking-droplet system with quantum pilot-wave theories, including those of Louis de Broglie and David Bohm, thereby providing a mathematical bridge between the macroscopic and microscopic realms. Finally, it has inspired the development of our Hydrodynamic Quantum Theory, an extension of de Broglie’s original theory informed by the hydrodynamic system.
- Generalized Pilot-Wave Framework: the free particle

- Hydrodynamic quantum field theory II

- Hydrodynamic quantum field theory I
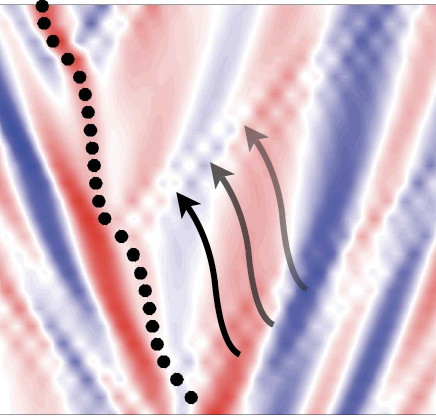
- Speed oscillations in classical pilot-wave dynamics
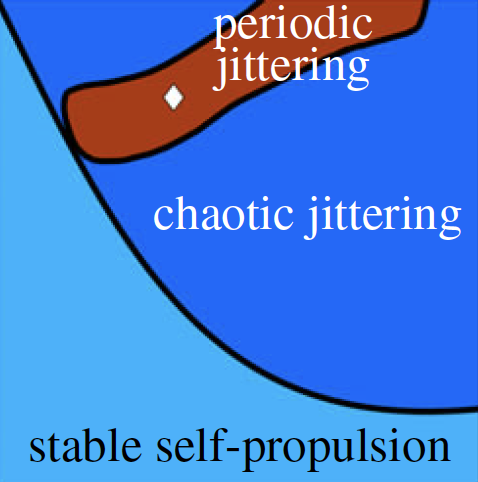
- Toward a generalized pilot-wave theory
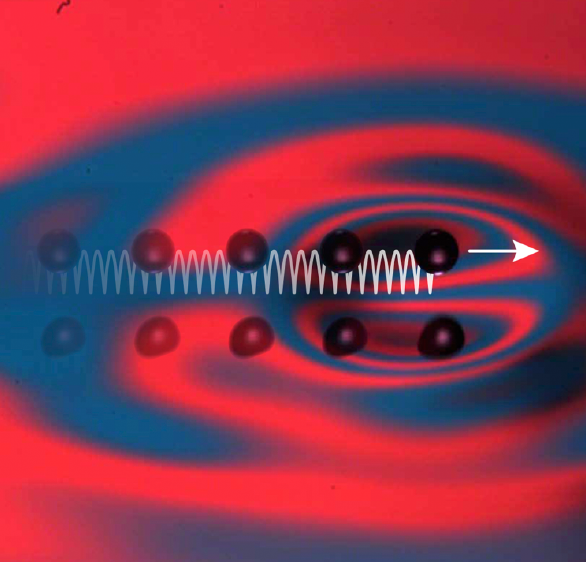
- Hydrodynamic spin states
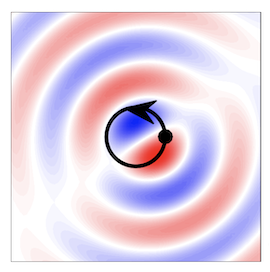
- GPWF: orbital dynamics and trapping
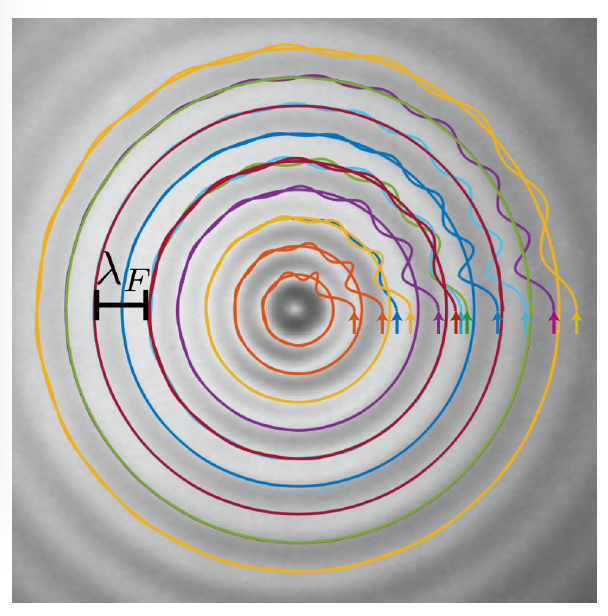
- Orbital dynamics: onset of chaos

