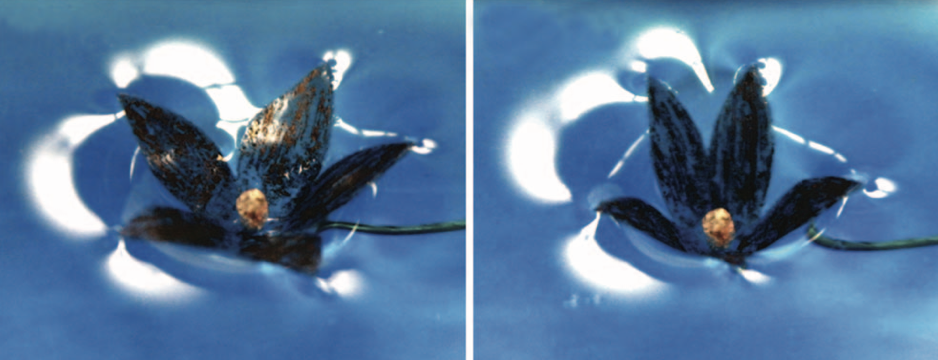
We consider the role of flexibility in the weight-bearing characteristics of bodies floating at an interface. Specifically, we develop a theoretical model for a two- dimensional thin floating plate that yields the maximum stable plate load and optimal stiffness for weight support. Plates small relative to the capillary length are primarily supported by surface tension, and their weight-bearing potential does not benefit from flexibility. Above a critical size comparable to the capillary length, flexibility assists interfacial flotation. For plates on the order of and larger than the capillary length, deflection from an initially flat shape increases the force resulting from hydrostatic pressure, allowing the plate to support a greater load. In this large plate limit, the shape that bears the most weight is a semicircle, which displaces the most fluid above the plate for a fixed plate length. Exact results for maximum weight-bearing plate shapes are compared to analytic approximations made in the limits of large and small plate sizes. The value of flexibility for floating to a number of biological organisms is discussed in light of our study.
See paper here: Burton & Bush (2012)
