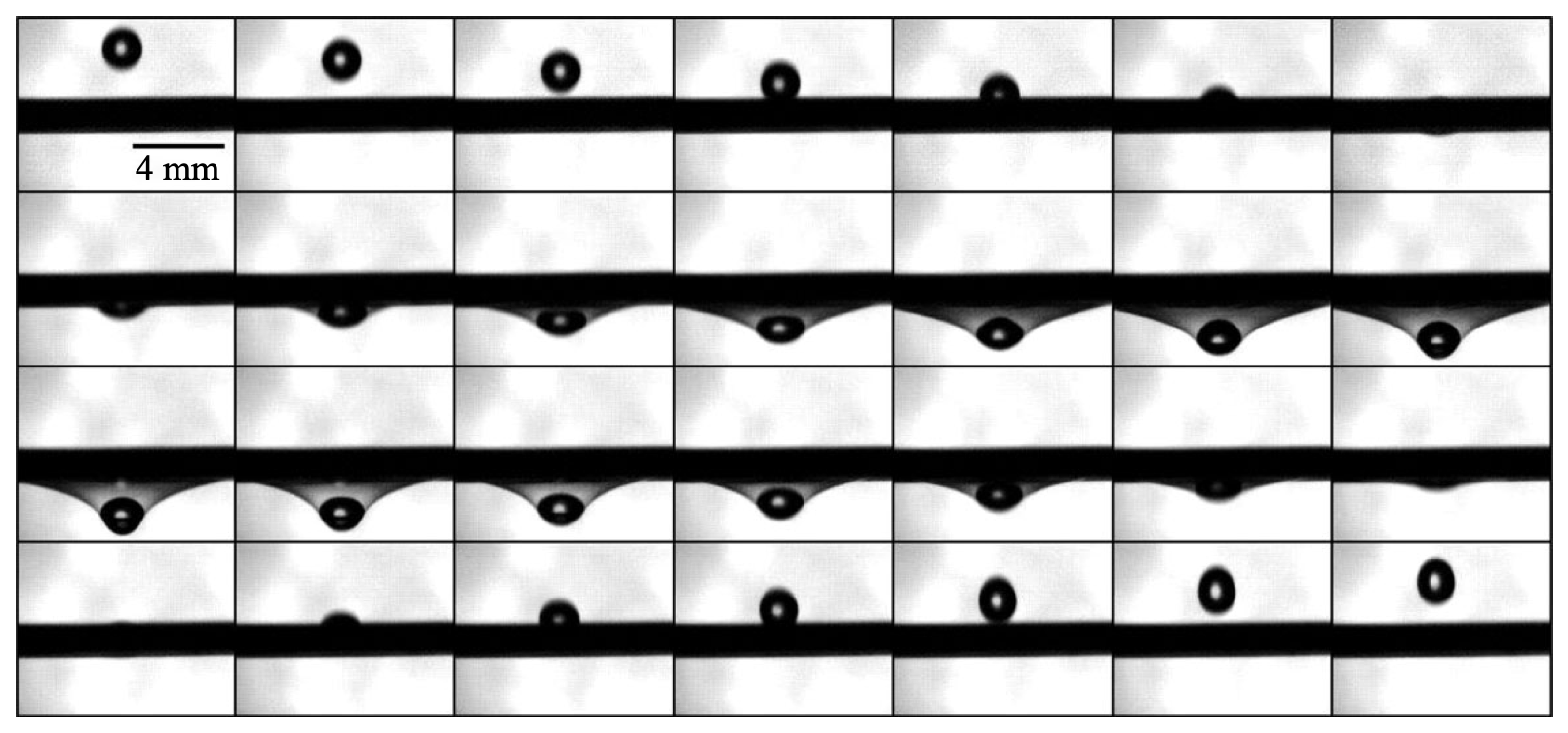
We examine the dynamics arising when a water droplet bounces on a horizontal soap film suspended on a vertically oscillating circular frame. A variety of simple and complex periodic bouncing states are observed, in addition to multiperiodicity and period-doubling transitions to chaos. The system is simply and accurately modeled by a single ordinary differential equation, the numerical solution of which captures all the essential features of the observed behavior. Iterative maps and bifurcation diagrams indicate that the system exhibits all the features of a classic low-dimensional chaotic oscillator. Our analysis of the dynamics was the first step towards a rational description of droplets bouncing and walking on a vibrating fluid bath.
See papers: Gilet & Bush (2009a) and Gilet & Bush (2009b) .
SELECT PRESS: MIT News , Scientific American , Nature Physics: News and Views