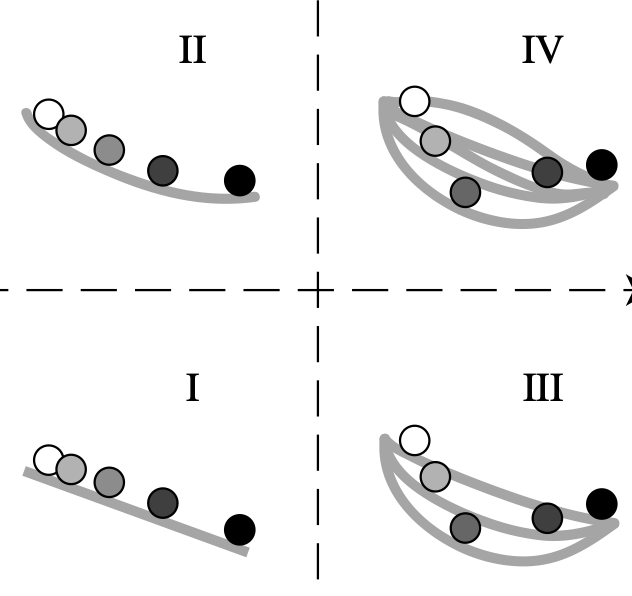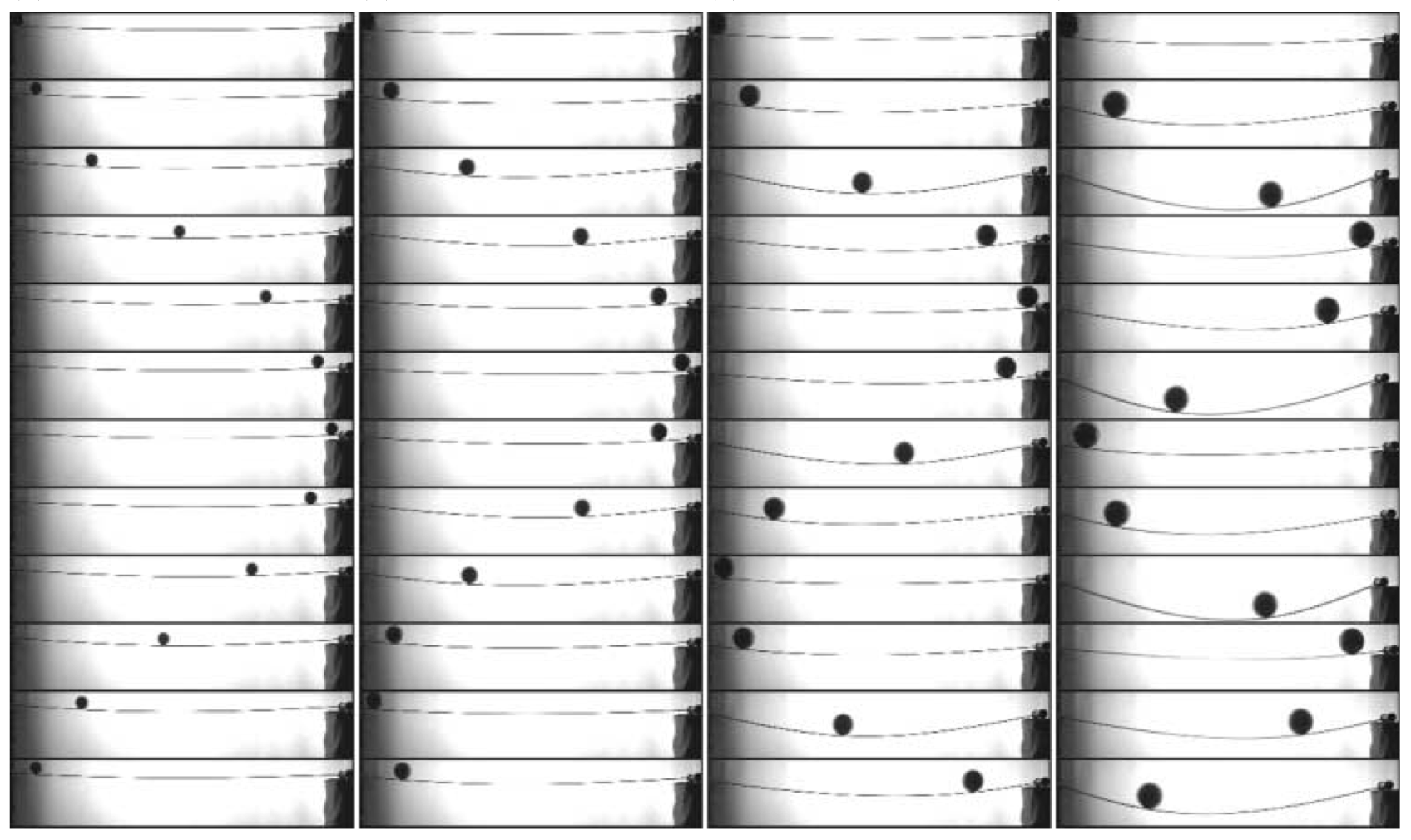
We present the results of a combined experimental and theoretical investigation of the motion of a sphere on an inclined flexible beam. A theoretical model based on Euler– Bernoulli beam theory is developed to describe the dynamics, and in the limit where the beam reacts instantaneously to the loading, we obtain exact solutions for the load trajectory and descent time. For the case of an initially horizontal beam, we calculate the period of the resulting oscillations. Theoretical predictions compare favourably with our experimental observations in this quasi-static regime. The time taken for descent along an elastic beam, the elastochrone, is shown to exceed the classical brachistochrone, the shortest time between two points in a gravitational field.
See paper here: Aristoff, Clanet and Bush (2009)