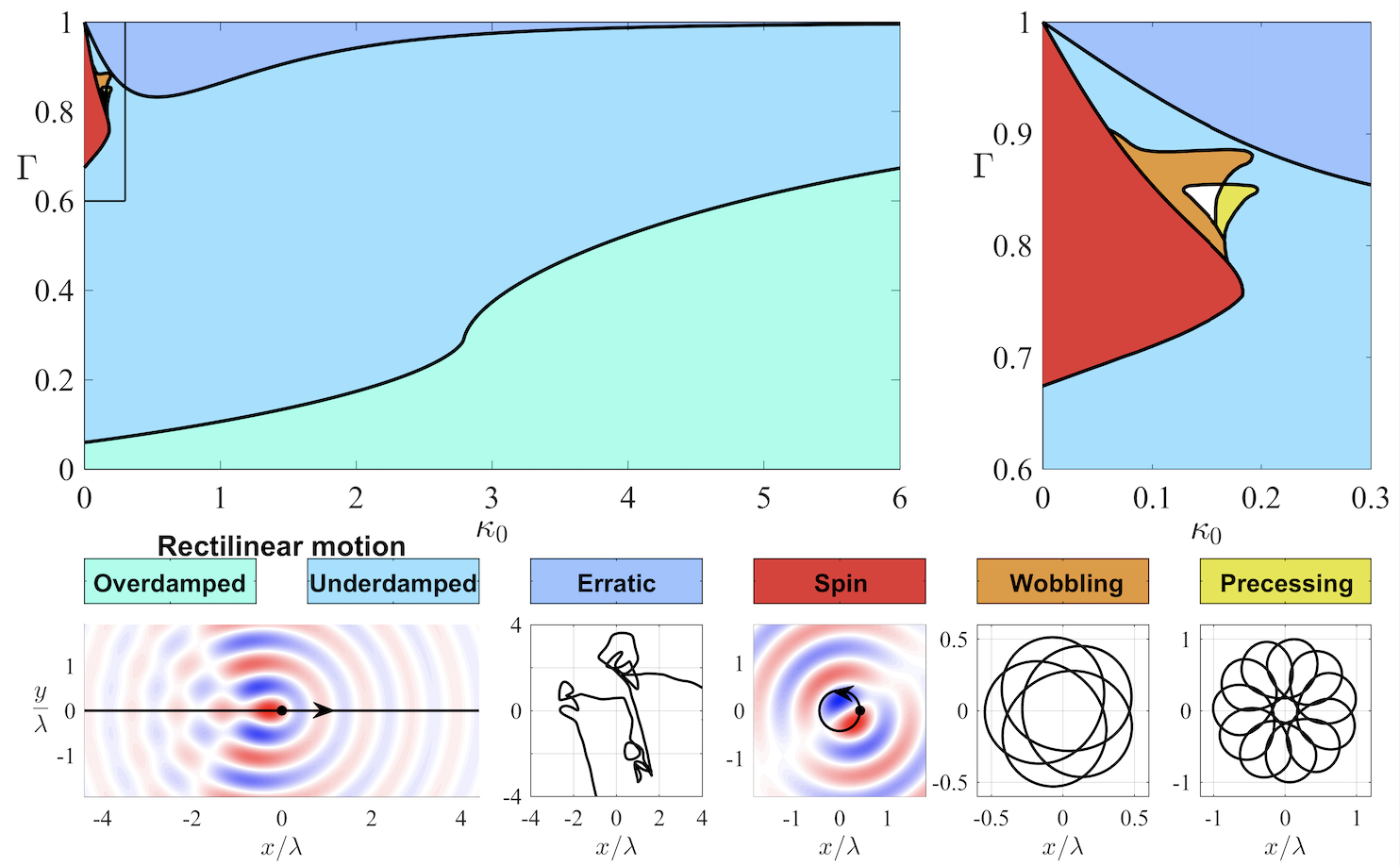
We present the results of a theoretical investigation into the dynamics of a vibrating particle propelled by its self-induced wave field. Inspired by the hydrodynamic pilot-wave system discovered by Yves Couder and Emmanuel Fort, the idealized pilot-wave system considered here consists of a particle guided by the slope of its quasi-monochromatic ‘pilot’ wave, which encodes the history of the particle motion. We characterize this idealized pilot-wave system in terms of two dimensionless groups that prescribe the relative importance of particle inertia, drag and wave forcing. Prior work has delineated regimes in which self-propulsion of the free particle leads to steady or oscillatory rectilinear motion; it has further revealed parameter regimes in which the particle executes a stable circular orbit, confined by its pilot wave. We here report a number of new dynamical states in which the free particle executes self-induced wobbling and precessing orbital motion. We also explore the statistics of the chaotic regime arising when the time scale of the wave decay is long relative to that of particle motion, and characterize the diffusive and rotational nature of the resultant particle dynamics. We thus present a detailed characterization of free-particle motion in this rich two-parameter family of dynamical systems.
See paper: Durey, M. and Bush, J.W.M., Chaos (2021)
Press: AIP Scilight
