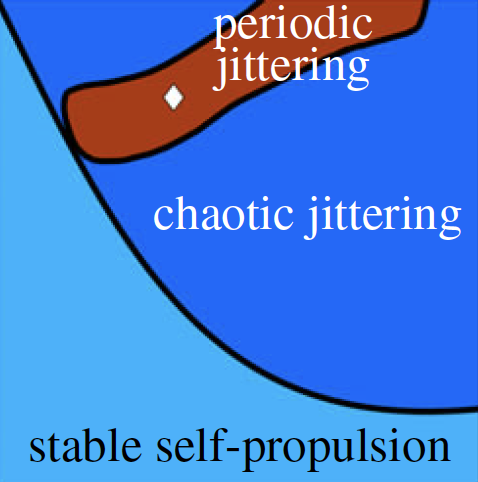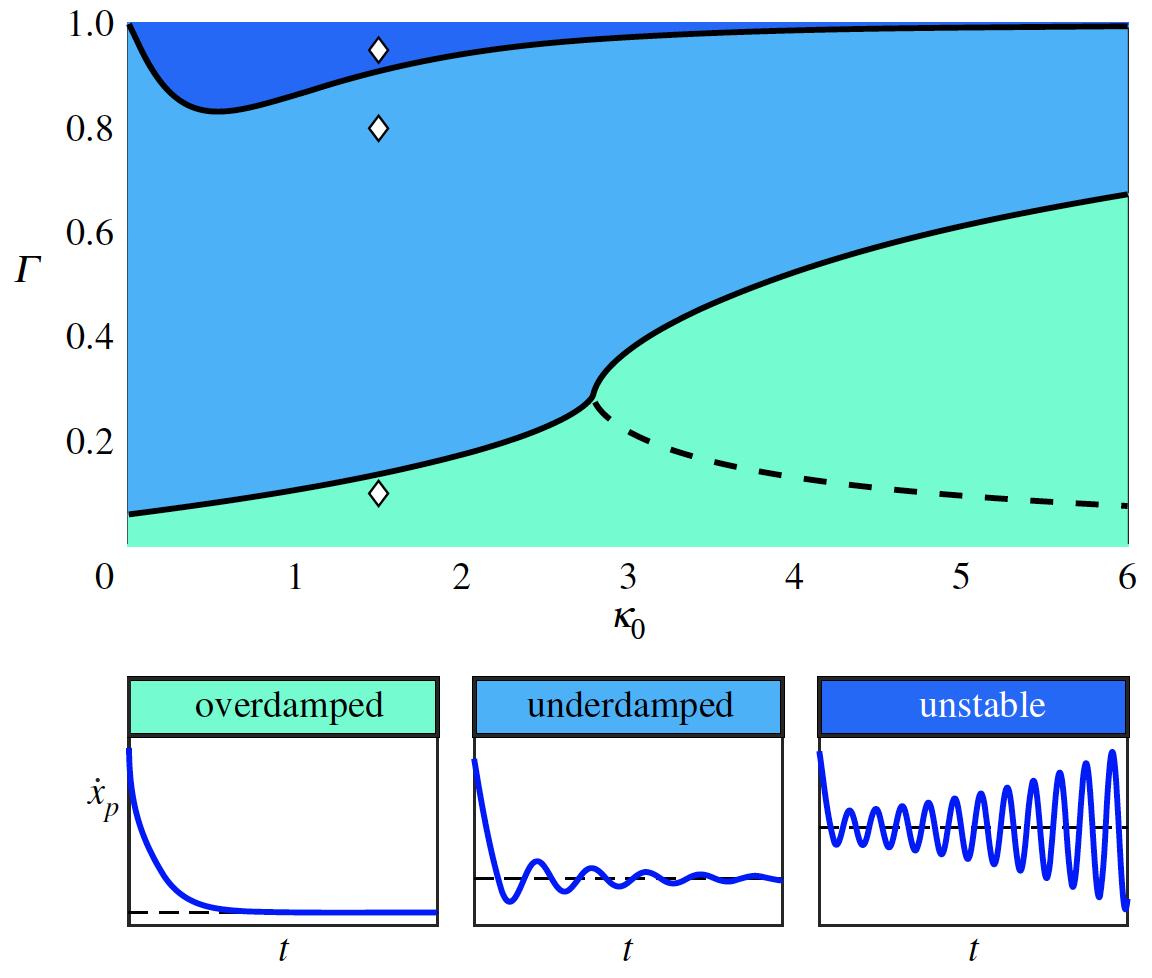
We present the results of a theoretical investigation of a dynamical system consisting of a particle self-propelling through a resonant interaction with its own quasi-monochromatic pilot-wave field. We rationalize two distinct mechanisms, arising in different regions of parameter space, that may lead to a wavelike statistical signature with the pilot-wavelength. First, resonant speed oscillations with the wavelength of the guiding wave may arise when the particle is perturbed from its steady self-propelling state. Second, a random-walk-like motion may set in when the decay rate of the pilot-wave field is sufficiently small. The implications for the emergent statistics in classical pilot-wave systems are discussed.
See paper: Durey, M., Turton, S.E. and Bush, J.W.M., Proc. Roy. Soc. A. (2020).